Neutron stars are so named because in the simplest of models they are made of neutrons. They form when the core of a large star collapses, and the weight of gravity causes atoms to collapse. Electrons are squeezed together with protons so that the core becomes a dense sea of neutrons. But we now know that neutron stars aren’t just gravitationally bound neutrons. For one thing, neutrons are comprised of quarks, which have their own interactions both within and between neutrons. These interactions are extremely complex, so the details of a neutron star’s interior are something we don’t fully understand.
The bulk properties of neutron matter are best described by the Tolman-Oppenheimer-Volkoff (TOV) equation of state. Based on this, the upper mass limit for a neutron star should be around 2.2 to 2.6 solar masses, which seems to agree with observation. The TOV equation also assumes that the neutrons within the neutron star remain neutrons. In atomic nuclei, you can’t have a sea of free quarks because of the nature of the strong nuclear force, so this seems like a reasonable assumption. But some physicists and astronomers have argued that within the dense heart of a neutron star, quarks might break free to create a quark star. Some have even suggested that quarks within a neutron star might interact so strongly that strange quarks appear, making them strange quark stars.
One way to explore these ideas is to look at pulsars. Since pulsars are rotating neutron stars where their magnetic pole sweeps in our direction, we can measure the rate of rotation by timing the radio pulses from a pulsar. So if a pulsar flashes every three seconds, we know that’s how long it takes for the neutron star to rotate once. Pulsars are how we first learned that neutron stars are, well, neutron stars, because the rate of an object’s rotation tells you the minimum density the object must have.
You can think of it like a playground merry-go-round. If you let a few children climb on, then spin the merry-go-round really fast, you can watch the kids fly off one by one as they lose their grip. This is one of the reasons playground merry-go-rounds are so rare these days. Since stars are held together by gravity, there is an upper limit on how fast a star can rotate. Any faster and gravity would lose its grip and the star would fly apart. So when we measure the rotation of a pulsar, we know it must be below that upper limit, known as the Kepler frequency. Since the surface gravity of a star depends on its density, the rotation frequency tells us the minimum density of the star. When astronomers first discovered pulsars rotating several times a second, they knew the density of the pulsar was greater than a white dwarf, so it had to be a neutron star.
There are some pulsars that have very high rotation frequencies. The fastest observed pulsars, known as millisecond pulsars, can have frequencies above 700 Hz. It’s pretty astonishing when you think about it. An object with nearly twice the mass of the Sun, but only a few kilometers across and making hundreds of rotations a second. Millisecond pulsars rotate so quickly that they aren’t even spherical. They bulge out around their equators to become oblate spheroids. This means the density in their polar regions must be much higher than near the equator. This raises the question of whether neutrons in the polar regions might undergo a phase transition into quark matter.
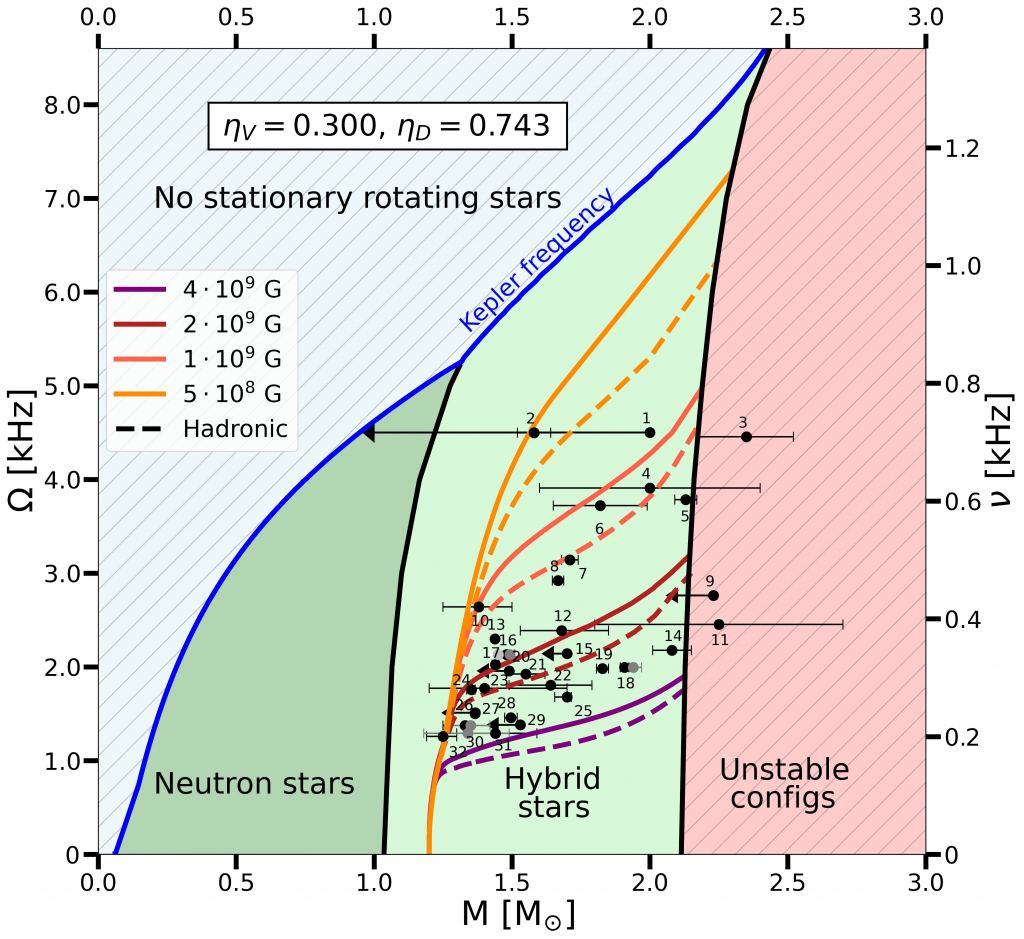
To explore this idea, a team looked at various models of neutron stars. They modeled the equation of state for traditional neutron stars and compared them to so-called hybrid stars, where the interior is a mix of neutrons and quark matter. From this, they calculated the Kepler frequency as it relates to the overall mass of the star. They found that while all the currently observed millisecond pulsars can be described by the traditional model, the hybrid model is a better fit for the fastest pulsars. They also calculated that hybrid stars would push the upper limit closer to 1,000 rotations a second. So if we find pulsars in the 800 Hz or higher range, we know they likely contain quark matter in their cores.
Another way to test the hybrid neutron star model would be to find more millisecond pulsars with a wide range of masses. This would allow us to look at how the rotation frequency varies with mass at the upper limit to see if Kepler frequencies agree more strongly with a hybrid or traditional model.
Reference: Gärtlein, Christoph, et al. “Fastest spinning millisecond pulsars: indicators for quark matter in neutron stars?” arXiv preprint arXiv:2412.07758 (2024).
Read our previous article: ‘Tragedy of the commons’ in space: We need to act now to prevent an orbital debris crisis, scientists say